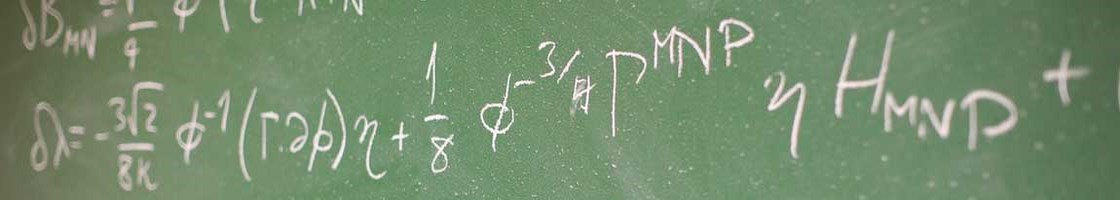What is Condensed Matter Physics and Topological Phases of Matter?
Condensed matter physics is a study concerned with the phases of condensed matter, most familiar are the solid and liquid phases of matter. There are however more exotic phases that include Bose-Einstein condensates, magnetic phases and superconductivity.
Research Topics:
The general properties of topological phases (using topological field theory) as well as models of systems with anyons and experimentally accessible systems such as the two-dimensional electron liquids of the fractional quantum Hall effect.
Our group is part of the Dublin Area Quantum Information Science and Technology group, which also includes members from Dublin Institute of Advanced Studies, University College Dublin, Trinity College Dublin and University College Cork.
People:
Dr. Masud Haque, Dr. Joost Slingerland, Dr. Jiri Vala, Dr. Brian Dolan, Dr Paul Watts, Dr. Babatunde Ayeni, Mr John Brennan, Mr Stephen Nulty, Mr Domenico Pellegrino, Mr Darragh Millar, Mr Aaron Conlon, Mr Phillip Cussen Burke, Mr Goran Nakerst, Mr Nathan Keenan, Mr Shane Short, Mr Gert Vercleyen
General Relativity
The force of gravity is universal on larger scales and governs the motion of planets and stars as well as the expansion of the Universe as a whole. The mathematical framework for describing the force of gravity when forces become large, close to neutron stars or black holes or at cosmological distances, is Einstein's general theory of relativity. General Relativity describes the force of gravity as being due to a warped geometry in four dimensions consisting of three space and one time dimension. The formation of black holes and the Big Bang 13.8 billion years ago are both encoded in Einstein's general relativistic equations which relate the geometry to the distribution of matter.
It was discovered by Stephen Hawking in 1974 that black holes behave as thermal systems, subtle quantum effects dictate that black holes radiate heat and the thermal properties of black holes is a current research topic.
When gravitational forces become really extreme, inside a black hole or during the first fraction of a second after the Big Bang, quantum effects become of paramount importance and Einstein's equations break down - we do not yet have a mathematical description of the physics in these extreme conditions. One proposal for a mathematical model that incorporate these effects is a modified geometry, called "non-commutative" geometry, based on a concept first introduced into mathematics by the Irish mathematician and physicist William Hamilton. Non-commutative geometry, both in General Relativity and in Quantum Field Theory is another research topic.
Cosmic Anomalies
The current standard cosmological model first most currently available cosmological observations, but some of this data suggests features that may need revisions or additions to the standard model in order to explain. Among the questions being addressed by this research are: is there evidence of departures from the standard model in the Planck observations of the cosmic microwave background; and is the apparent tension between different determinations of cosmological parameters (e.g. the Hubble constant) from different data sets.
Wave Mechanics and Large-scale Structure
This work investigates the application of an idea originally suggested by Widrow & Kaiser that involves representing the large-scale distribution of matter using a wave-mechanics, specifically using the Schrödinger-Poisson description. This approach has numerous technical advantages over the standard methods but is far less widely studied. We will be applying it to problems involving dark matter in the form of ultra-light particles as well as the problem of redshift-space distortions and velocity-density reconstruction.
Euclid
The European Space Agency's Euclid mission , scheduled for launch in mid-2022, is
intended to better understand dark energy and dark matter by accurately measuring the acceleration of the universe using a number of complementary approaches. Prof. Coles is a member of the Euclid Consortium in which he participates in the Science Working Group on Galaxy Clustering, which is currently preparing for this launch. The analysis of data from Euclid will take many years after the survey is itself completed so this will become a main focus in the medium to long term.
People:
Prof. Peter Coles, Prof. Brian Dolan, Dr. John Regan, Mr. Aonghus Hunter-McCabe
General Relativity and Quantum Gravity
The force of gravity is universal on larger scales and governs the motion of planets and stars as well as the expansion of the Universe as a whole. The mathematical framework for describing the force of gravity when forces become large, close to neutron stars or black holes or at cosmological distances, is Einstein's general theory of relativity. General Relativity describes the force of gravity as being due to a warped geometry in four dimensions consisting of three space and one time dimension. The formation of black holes and the Big Bang 13.8 billion years ago are both encoded in Einstein's general relativistic equations which relate the geometry to the distribution of matter.
It was discovered by Stephen Hawking in 1974 that black holes behave as thermal systems, subtle quantum effects dictate that black holes radiate heat and the thermal properties of black holes is a current research topic.
When gravitational forces become really extreme, inside a black hole or during the first fraction of a second after the Big Bang, quantum effects become of paramount importance and Einstein's equations break down - we do not yet have a mathematical description of the physics in these extreme conditions. One proposal for a mathematical model that incorporate these effects is a modified geometry, called "non-commutative" geometry, based on a concept first introduced into mathematics by the Irish mathematician and physicist William Hamilton. Non-commutative geometry, both in General Relativity and in Quantum Field Theory is another research topic.
Nonperturbative Quantum Field Theory
Understanding the strong interactions, the interactions of quarks and gluons. In ordinary conditions, quarks and gluons can never exist as free particles, but are instead confined within composite particles such as protons and neutrons. At extremely high temperatures or densities, which existed in the early universe and may exist in the cores of neutron stars, it is predicted that quarks and gluons can be liberated, forming new states of matter called the quark-gluon plasma or quark matter.
It is only at extremely high energies that standard perturbative methods can be applied to the strong interactions. At low and intermediate energies, the theory can be defined by discretising space and time to form a lattice, and studied by computer simulations.
Confinement of quarks and gluons is still far from understood. One way of approaching this issue is by studying the properties of the quarks and gluons themselves and how they couple to each other at different energy and momentum scales. Signatures of confinement will show up in the infrared régime or quark and gluon correlators. The same forces that produce confinement also give rise to about 98% of the mass of everything we see around us.
Research Topics:
Experiments at Brookhaven and CERN are currently trying to produce the quark-gluon plasma and study its properties. Major theoretical issues surrounding this include: at what temperature does the deconfinement transition happen, what is the energy density, pressure and viscosity of the plasma, which bound states survive, and what are the properties of the deconfined quarks and gluons.
At high density, quark matter may form a number of exotic superconducting phases. Lattice simulations encounter serious problems in this régime, but insight may be gained from similar theories which do not suffer these problems, such as Quantum Chromodynamics with 2 colours instead of 3. Results from such simulations may also be used as input or controls for model calculations.
People:
Dr. Brian Dolan, Dr. Jonivar Skullerud, Dr. Paul Watts
What is Nonlinear Dynamics?
The study of nonlinear dynamics is a study of differential equations and difference mappings, with variables whose changes can not be captured with a linear combination of variables. Simple changes of an input variable in such systems or small changes in a systems parameters can lead to, directly, disproportionate changes in the system of study. Phenomena such as Chaos and the Butterfly Effect can be found in nonlinear dynamical systems.
Research Topics:
The Nonlinear Dynamics and Chaos Group is interested in developments in nonlinear dynamical systems theory, multifractal analysis, bifurcation categorisation, quantum chaos and applications of nonlinear dynamics to biomedical systems (such as Cardiac and Neural networks) and models of Financial Markets. The underlying focus is on capturing universal properties of nonlinear systems that are applicable to a large class of problems which exhibit nonlinear phenomena.
People:
Prof. Daniel M. Heffernan